Pyritohedral hypergon in blue and goldさんのイラストまとめ

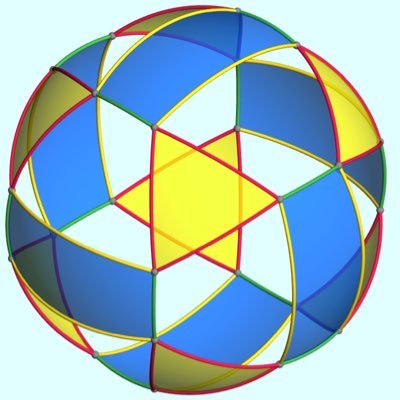
Day 28, #Maydala, an exploration with a reflexed octagon.

Day 11 #Maydala, a celtic knot pattern 8-petal flower weaved as 8-3 octagram, with a ring.
Polytile: 128:-16.(1^12).-4.(.-10^8).-4.(1^12)^8, with circle, with path line widths added for under/over.

@becky_k_warren Very nice Becky! I see it can be seen as a compound of 2 6-sided stars.

@AlbertPCarpent1 @maanow @amermathsoc A fun pattern! I wonder what comes next?

Another offset square tiling makes a weave pattern (left), while duality applied twice puffs them out into heptagons and squares, which can seen as a tetra-truncated Cairo pentagonal tiling.
https://t.co/YAz7GwERgP

@amirvaxman_dgp I have a Java application I wrote that edits 2D geometry, vertices/edges/polygons, and recently added an equilateral tile option I call polytiles. Not much UI for new code, but I can do a lot with it pretty quick, like removing vertices/edges here.

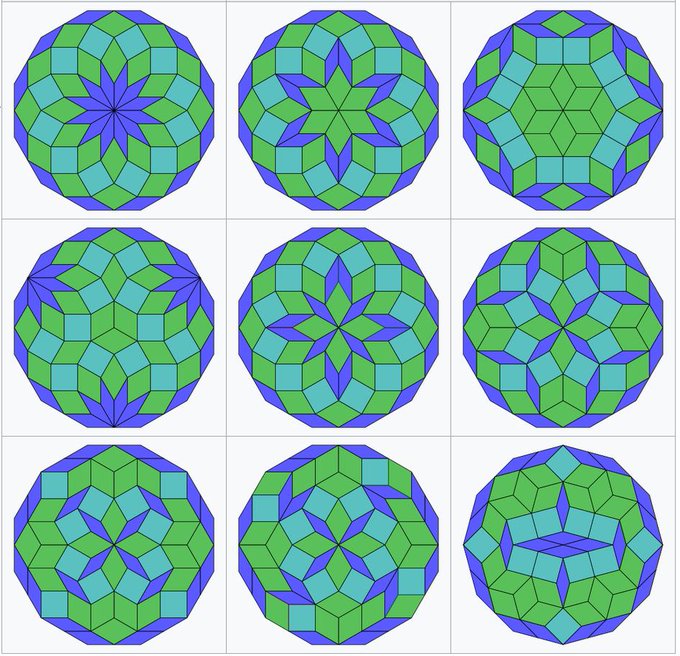
A dodecatile rosette centered on a star: dodecus star -3.4^12, square 3^4, hexare 2^6, thin rhomb 1.5^2, wide rhomb 2.4^2, and flat octagon 1.1.1.3^2.

This wild decatile, -1-133-1-13113, is a decagon with 2 hexagons removed. Hard to see what it can do in this random tiling, but almost seems like it could be a puzzle of sorts, where a free decagon can be rotated, leading to other free decagons propagating. Too random for me.

@becky_k_warren @Simon_Gregg @Trianglemancsd A few symmetric solutions on wikipedia.
https://t.co/68sMilP8jC